How Polaris Can Be Used To Confirm A Spherical Earth
This is a variation on the Simple proof of Earth curvature, but using Polaris (Alpha Ursae Minoris) as our fixed point. You'll recall we've also done this with a mountain peak.
But it all works for the same simple geometric fact - angles to some fixed point behave differently with distance on a curved surface versus angles on a flat surface.
First of all when we look at Polaris what are some of the things we can (hopefully) all agree upon?
Observations
- Polaris doesn't move much in the sky (it has a 40' offset from dead center)
- If you looked straight up from the North Pole (give or take), Polaris would be overhead
- At 45° North latitude Polaris is at ~45° Altitude (varies slightly from 45°±40')
- At the Equator, Polaris is on the horizon (varies from 0°±40')
I've seen Flat Earthers CLAIM that Polaris can be seen far to the South of the Equator, they are wrong. When you check into their claim they are wrong 100% of the time. Usually because they try to deny that their is a South pole star so they get Southern star trail photos mixed up with Northern star trail photos. If you believe you can see Polaris from ground level at more than a few degrees South of the Equator (because it's 40 minutes above the Horizon at peak right now) then you'll need to present your evidence to be evaluated.
Given our starting observations let's consider the following diagram...
ON A FLAT PLANE
Given our starting observations let's consider the following diagram...
 |
| Figure 1 - angles on a plane would never reach zero |
 |
| Figure 2 - twice the distance gives one-half the slope |
IF the ground was flat this would imply that Polaris was 3097.5 miles above the Earth and that if we move another 3097.5 miles south to the Equator (point Z) then we should see Polaris at a slope of 0.5 which is equal to an angle of 26.565° as given by tan⁻¹(0.5).
Regardless of how far South you think you can see Polaris it is trivial to confirm that it is no where even near 26.565° above the Horizon.
This just doesn't match reality. Sorry Flat Earth.
Saul Trane's excellent post on Polaris shows that if we plot the angles to polaris at various latitudes we get nonsense:
 |
| Image Credit: Debunking the Flat Earth |
ON A CURVED SURFACE
How does this compare to what we expect on the curved surface of the Earth?
On a curved surface our horizon is constantly rotated so our APPARENT angles get smaller faster, and eventually would go negative.
 |
| Figure 3 - green line marks our horizon as rotated 45°, so 45° off the horizon is actually straight up relative to the pole/equator |
Since Polaris is very distant the angular shift over the distance from our North Pole to the Equator is imperceptible and can be ignored. This is why we can simply measure the angle of Polaris above the Horizon and find our latitude (±40' due to Earth's rotation and the offset).
And this is what we find, which comports with reality.
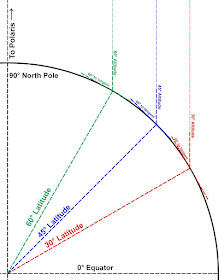 |
| Image Credit Unknown |
Polaris
Polaris is a fairly bright (Magnitude 1.95) star that just happens to be near the North celestial pole right now.
However, Polaris doesn't remain in a fixed position near our North celestial pole due to precession of Earths' axis it swaps out with Vega in a cycle that takes 26,000 years to complete. So while it does move it moves very slowly. Indeed we can compare the position in 1995 with the position in 2016 and see that it's shifted very slightly but measurably over those 21 years.
The field "RA/DE (of date)" gives you the Right Ascension and Declination of the object, the Declination is all we need to consider as that is how many degrees the object is away from the celestial equator at 0° and the North Celestial Pole is at 90°.
These are just snaps from the app Stellarium - for confirmation you'd need to talk to an Astronomer and get access to historical data. If I dig some up later I'll add them here (or you can post as comments and help me out).
 |
 |
| Polaris: 1995 | Polaris 2016 |
A diagram to help understand how Right Ascension and Declination work...
 |
| Figure X - Credit WikiMedia |
This image shows the circular arc of Polaris, taken from Vienna. It was created by taking a partial star trail and completing the rotation digitally so you could see the full circle. Unlikely many star trails this photo also has a fairly narrow field of view (telephoto) so the arc of Polaris can be seen very clearly.
 |
| Star Trails Over Vienna Credit & Copyright: Peter Wienerroither (U. Wien) |
No comments:
Post a Comment
Note: Only a member of this blog may post a comment.